ESP32简单OTA升级
本文共 269 字,大约阅读时间需要 1 分钟。
开发环境: esp-idf-tools-setup-2.3.exe,esp-idf-v4.1,python3.6.2
在开发环境搭建中遇到一些问题,主要是国内访问github.com速度很慢导致下载一些依赖文件无法成功。
在ESP32 simple_ota_example案例中,使用https升级,折腾好几个晚上都没有升级成功,所以考虑使用http升级。
使用http升级可以很简单的升级成功,现就通过http升级成功过程说下。
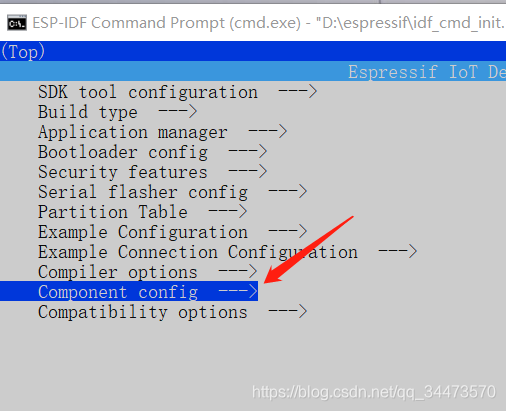
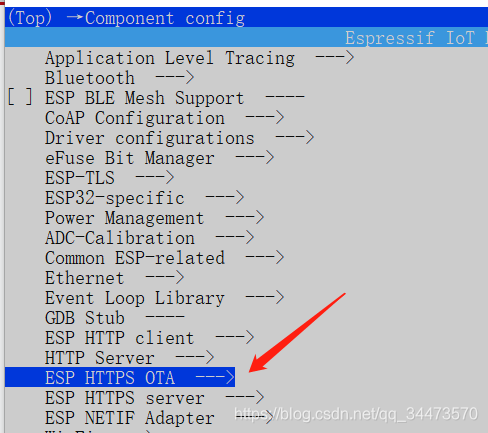

选择使用http方式升级。

之后就可以烧录,在要升级的固件目录中开启http server就可以正常访问。
转载地址:http://czer.baihongyu.com/
你可能感兴趣的文章
Mysql之备份与恢复
查看>>
mysql之子查询
查看>>
MySQL之字符串函数
查看>>
mysql之常见函数
查看>>
Mysql之性能优化--索引的使用
查看>>
mysql之旅【第一篇】
查看>>
Mysql之索引选择及优化
查看>>
mysql之联合查询UNION
查看>>
mysql之连接查询,多表连接
查看>>
mysql乱码
查看>>
Mysql事务。开启事务、脏读、不可重复读、幻读、隔离级别
查看>>
MySQL事务与锁详解
查看>>
MySQL事务原理以及MVCC详解
查看>>
MySQL事务及其特性与锁机制
查看>>
mysql事务理解
查看>>
MySQL事务详解结合MVCC机制的理解
查看>>
MySQL事务隔离级别:读未提交、读已提交、可重复读和串行
查看>>
MySQL事务隔离级别:读未提交、读已提交、可重复读和串行
查看>>
mysql五补充部分:SQL逻辑查询语句执行顺序
查看>>
mysql交互式连接&非交互式连接
查看>>